A New Way to Help the Technical Library
We are very grateful to the people who have donated to the technical library over the years. The cost to keep it going is significant and we appreciate every contribution no matter how small.
However, donating is difficult as you are making a cash gift for nothing tangible in return (OK, you get some stuff from the website, but there is a tenuous physical relationship there….)
In order to make it easier for people to support the technical library we have moved from asking for donations to selling engineering related products. You can see what we have on offer here.
Our intent is to make the designs relevant to engineers, unique and amusing where we can.
I have ordered some of the products as samples and I am happy with the quality and the value and am going to treat myself to a number of the ‘bad patent’ t-shirts and sweaters.
We will be updating the range of merchandise and the designs so please do check out the store on a regular basis and if you can make your engineering colleagues and friends aware I am sure they will find something that will amuse and delight them.
May
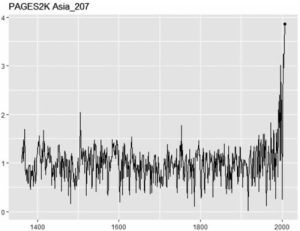
After a particularly cold and dull May here in the UK we were informed with an undeserved level of confidence that this was the Hottest May on record since records began.
I immediately realized that my thermal underwear might be exposing me to dangerous levels of UV radiation so I immediately swapped them for a thick coating of sunblock. I turned off the heating, huddled in a corner, and let the global warming freeze me gently to sleep.
Here is the exciting news:
When this was widely challenged we were told that it was hotter at night and that is why nobody noticed.
We spent May with the winter blankets on the bed. So it was a record-setting May not only because the heat snuck in while you were sleeping, it also snuck in at night everywhere else and just avoided my bedroom and probably your bedroom too. Darn it – just our luck.
And I completely believe that. Because when you search for the data you get a summary from the UK government that shows that the temperature has been inexorably increasing because of all the SUVs and the other icky stuff.
You know you can trust it because there is no reference given to the source data. The results are so accurate and certain they require no citation. The data would only confuse you, peasants. The political summary is what you are interested in. Inquiry leads to questions, questions lead to uncertainty, uncertainty leads to sadness and sadness leads to doubt and doubt means you have a lack of faith, sinner!
In 2004 the method for generating the current and historical climate data in the UK was changed. The new method of generation is defined here:
It is worth scanning through these types of documents to get a feel for how the data is processed. What you see in published climate data is not scientists looking at the temperature records and taking a simple average. There are methods for eliminating station data from the values calculated. There are naughty stations.
At this website:
https://www.metoffice.gov.uk/research/climate/maps-and-data/data/haduk-grid/methods
You can read this:
“The gridded data sets are based on the archive of UK weather observations held at the Met Office. The density of the station network used varies through time, and for different climate variables — for example, for the temperature variables the number of stations rises from about 270 in the 1910s to600 in the mid-1990s, before falling to 450 in 2006.”
The number of stations used as contributing data points to the overall dataset is highly variable over time (that should give you confidence in the historical record!). This variability is not only because these stations no longer exist. It is because their data is excluded from the overall record.
There is a methodology for excluding stations and filling in the gaps in the data. The fewer stations included the more ‘derived’ data is used to fill the gaps.
Typically you do this in a statistical study to eliminate results that are likely errors and are not statistically valid. Of course, you can select what qualifies as outlying data. Good luck finding out which stations were omitted and why.
When we look at the temperature record what we are seeing is a post-processed set of data from a subset of overall weather stations, selected with an unknown methodology, supplemented with ‘data’ created to fill in the gaps. This can be valid, but it can also create a false impression of a lack of scatter, or false determinism and hide real uncertainty in the dataset. In the worst case, it can bias the dataset in one direction. Of course, I would never suggest that is what is being done on purpose.
Should you trust government climate data? You should trust it as much as you trust any other government-produced metric. Inflation, unemployment, migration, GDP etc. It has the same credibility.
Was May 2024 the warmest May in 150years in the UK?
Heh.
